Kaleidoscopes work the way they do because of a series of mirrors placed inside the tube. By changing the number of sides and the angles of the mirrors, artists are able to create a virtual never ending variety of images in their kaleidoscopes.
Three-mirror systems have, as you would guess, three mirrors, which are arranged in a triangle. This causes an endless field of patterns that continues up the whole tube of the kaleidoscopes.
One can see an example of a three-mirror mandala at left in the Small Classic Agate kaleidoscope by artist Jon Greene of Chesnik Scopes.
Most three-mirror systems are arranged in an equilateral triangle, creating an even, six-pointed image. Instead, some kaleidoscopes with three mirrors are formed into an isosceles triangle of mirrors, creating the same field of patterns, but with a larger number of points to their mandalas or interior images. This is sometimes called an expanded two-mirror system.
One can find a three-mirror, 8-point mandala pictured at right by artist Jon Greene of Chesnik Scopes.
Peggy and Steve Kittelson of Woodland Designs have added an interesting effect to their "Sparky" kaleidoscope, pictured at left, by drawing a black line along the "seam" between two sides of the mirror creating a fascinating, radiating effect.
Another way to change a three-mirror kaleidoscope is to taper the mirrors, from large to small, giving the image a 3D, "disco ball" effect. An example is the "Baby Planet" kaleidoscope by David Sugich, pictured at right.
Two-mirror systems use a triangle of mirrors, but one side is blacked out, making a single, circular design which is dramatically surrounded by black. Depending on the angle of the mirrors, the image can have 4, 5, 6, all the way up to over 100 points!
Some of our most popular two-mirror, 7-point mandalas are evident in the Tidepools line of kaleidoscopes by artists Luc and Sallie Durette of Durette Studios, pictured at left.
Some artists experiment with the non-mirror side of their two-mirror systems. Pictured to the right is Thomas and Carol Paretti's "Tilt-a-Whirl" where they used a scalloped piece of wood, instead of simply blacking out the third side to outline the mandala. That scalloped wood is then reflected onto the other two sides of mirror, creating a scalloped view all around.
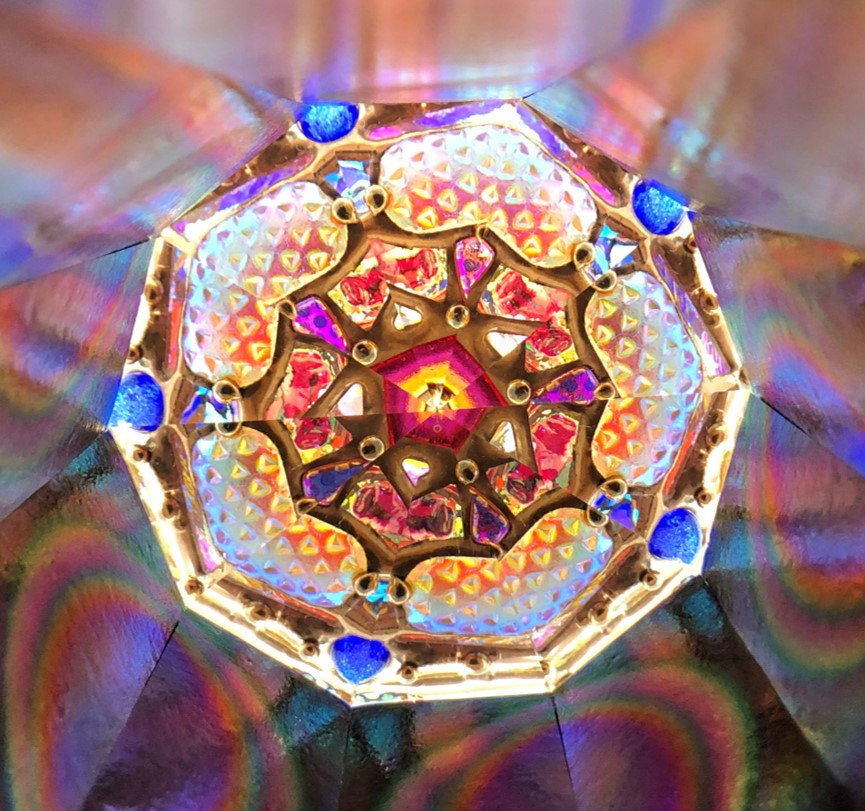
On the left is an image from Sue Rioux of Sue Rioux Designs' magnificent "Wishes" kaleidoscope, where the third side of mirror has been replaced with highly reflective dichroic glass. On the right, Steve and Peggy Kittelson of Woodland Designs have also replaced the third side of their mirror system with dichroic glass, creating a beautiful mandala.
Four-mirror systems which are arranged in a rectangle create a "parade" of images. The four-mirror rectangular mandala pictured at right is by artists Steve and Peggy Kittelson of Woodland Designs.
Four-mirror systems which are arranged in a diamond shape, also known as twin two-mirror systems, create an image with multiple focal points.
Four-mirror diamond mandala pictured at left is by artist David Sugich of Ultimate Reflections.
Corki Weeks also has a kaleidoscope with a particularly unique view. Her "Tango" kaleidoscope, pictured at right, features a modified 4-mirror system. Two of the four sides of mirror have a fixed metallic design, leaving two left to give a "chorus line" effect.
Kaleidoscopes with multiple mirror systems have more than one eyepiece showing different mandalas.
Artist Sue Rioux is well known and admired for her many styles of kaleidoscopes that feature two different mirror systems in the same kaleidoscope. One side will show a two-mirror system, while the other will show a three-mirror system. One example is the "Blue Raspberry" kaleidoscope, shown at left.